Ferrite core loss in HF power applications

For broadband transformers in the high frequency range, the only really usable core material is ferrite. Over the years several manufacturers have developed and produced ferrite materials of various permeabilities that can be used in HF power transformers, such as transmission antenna baluns, power amplifier coupling and matching, or hybrid combiners, but the manufacturers seem to have striken a complot about not to publish the loss data for their cores! While they do publish loss data for low-frequency cores made for switching power supplies, the data sheets for almost all of their HF cores do not include loss data for the radio frequency range. This forces circuit designers to follow very imprecise rules of thumb, and then experiment to find out whether the transformers will have acceptable loss.

For several decades I designed my RF transformers in this way, but now (2019) I thought it was time to do something about it. So I spent a good week of my time measuring the loss of 10 different ferrite cores, in the frequency range of main interest to radio amateurs, that is, 1.8 to 54MHz, at flux densities in the range that would be used in actual designs. This page presents the results, and my comments about them.

The method

Each core under test got a winding having a number of turns that would allow driving the core to the desired level. This winding was fed from an HF transceiver, using an antenna tuner when necessary, the coax cable then going on to a dummy load. A 100:1 high voltage scope probe connects to the winding, to measure the applied voltage. The 100:1 ratio is necessary to keep the probe from burning out, since 10:1 probes normally don't survive enough RF voltage to use them in this test. But the 100:1 ratio is also advantageous in that the input impedance of such a probe is higher than that of a 10:1 probe, reducing the effect of the inductance of the probe tip and the grounding clip and wire.
  
A temperature sensor was attached to each core under test, by means of kapton adhesive tape and a small amount of thermally conducting grease.

For each mesurement the core was wrapped in cotton, to serve as thermal insulation. Some time was allowed for the temperature to stabilize, then a specific RF voltage was applied, calculated to produce the desired flux density in the core, whose dimensions were accurately measured. After a certain time, typically 3 minutes, the drive signal was shut off, and again some time was allowed for the temperature to stabilize. The temperature rise was used together with the heat capacity of the core to calculate how much energy had been lost as heat, and this value was divided by the core volume to get the power loss per cubic centimeter of core material.

After each measurement the cotton was removed, and a fan was used to cool the core back down to room temperature. The average total time needed for each measurement was around 15 minutes, adding up to several hours per core.

The test frequencies were the low band edges of each of the amateur bands related in approximate or exact 1:2 ratio: 1.8, 3.5, 7, 14, 28 and 50MHz. On each frequency two tests were done, at flux densities having a 1:2 ratio. The voltage was calculated for each core, so as to give the same two levels of flux density in all cores. The drive voltage was kept constant on all frequencies for each of the two levels, so that the flux densities decreased in inverse proportion to frequency. This reflects exactly how such cores get used in many practical situations: At constant voltage and constant power throughout the frequency range.

 In some cases some additional measurements had to be done at additional levels of flux density, when one of the two main measurements was in a range where precision suffered.

The results

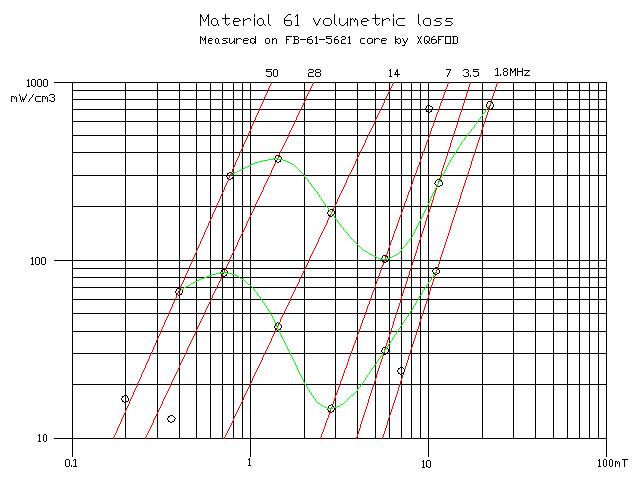The first core I tested was an FB-61-5621. This is a medium size cable bead made of the material most commonly used by hams for low loss RF power transformers. It has an initial permeability of 125. The part number is the one used by Amidon, the distributor where I bought it. Fair-Rite, the company manufacturing these cores, uses different part numbers.

The little circles in the graph represent the actual measured values. I drew the red loss curves as straight lines across the two measurements that I thought are the most relevant. The manufacturers mostly draw them as straight lines too, for the ferrites for which they do give such curves, but I would be very surprised if these should truly be straight lines! But drawing these curves as they really are, slightly bent, would require at least 4 to 5 measurements per frequency, and I didn't want to spend the rest of my life measuring ferrite loss...  

It's important to keep in mind, then, that in my graphs the parts of each line that lie between the two measurement values used to draw it, and close to them, are the most accurate, while any extensions of those lines into a range where I made no measurement becomes increasingly inaccurate. The important point here is that my measurements cover the levels of flux density most likely used in practice, so that these graphs can be used quite well for design.

The two green lines directly represent the volumetric loss of the ferrite through the spectrum, at the two fixed drive levels. The upper green line always represents a drive level such that a flux density of 22mT results on 1.8MHz, and the lower green line is at half that flux density. So these green lines allow directly comparing the loss of one ferrite to that of another. This is much easier to visually interpret, than the red loss lines per frequency.
  
61 ferrite turned out to have a very idiosyncratic loss curve, with a very low loss at 7MHz, the loss rising toward both higher and lower frequencies, but dropping again when going beyond HF into the low VHF range. I won't risk any explanation for this behaviour; I just present the facts, as measured.


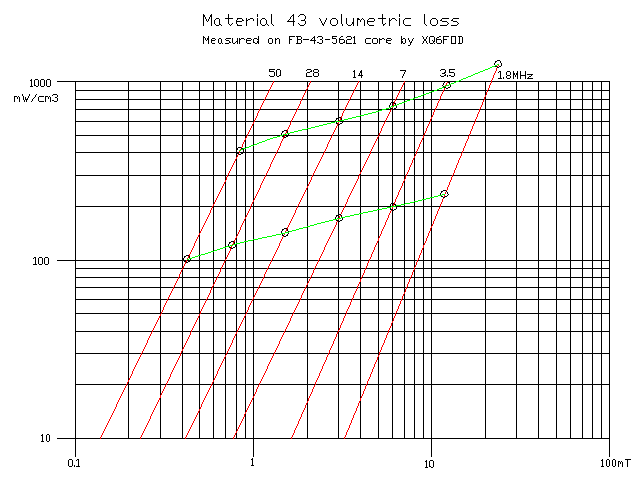The next core I measured was of the same nominal size, but made from material 43, which has a much higher initial permeability of 850.

The behaviour of this material was extremely smooth, even, predictable, almost boringly so. This looks nice at first sight - but the loss was also higher than that of the 61 material, on all frequencies!
 
Note that at high flux density on 1.8MHz, the loss of material 43 turned out only out 60% higher than that of material 61. But on 7MHz, where 61 has its sweet spot, 43 is 7 times more lossy than 61, at high flux density, and a whopping 15 times more lossy than 61 at moderately low flux density!


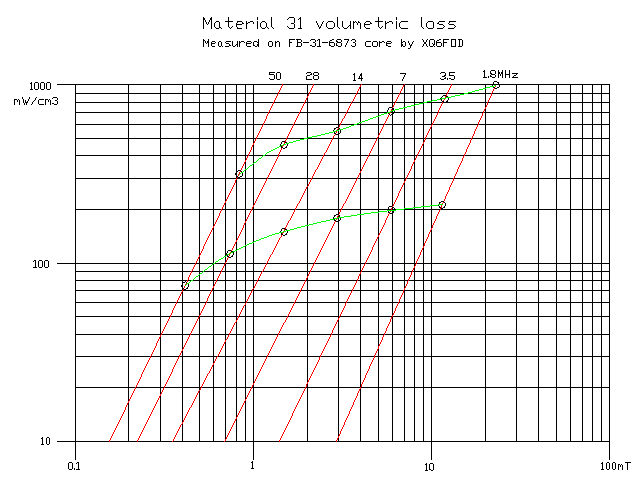A lot has been written in the last few years about material 31. Most RF cores are made from nickel/zinc ferrites, while low frequency cores are manganese/zinc ferrites. Manganese/zinc ferrite has fundamentally higher permeability, its loss increases less with flux density than in nickel/zinc ferrites, but it's also much more conductive than nickel/zinc, and has far lower velocity of propagation, which leads to dimensional resonance problems at RF. These two latter characteristics make most manganese/zinc poor for RF transformers.

But all the characteristics of a ferrite material depend not only on its basic formulation, but also on many details of the manufacturing process, over which the manufacturer has ample control. And so the Fair-Rite company came out with this material 31, which is an RF-suitable manganese/zinc material having a permeability of 1400, which is very high for RF use. I bough a small selection of material 31 cores, to try this material.
  
The results show that the material very much resembles 43, in terms of loss. The difference in loss is too slim to be enough reason to choose between 31 and 43. But where its higher permeability is an advantage, 31 is really the material to use.


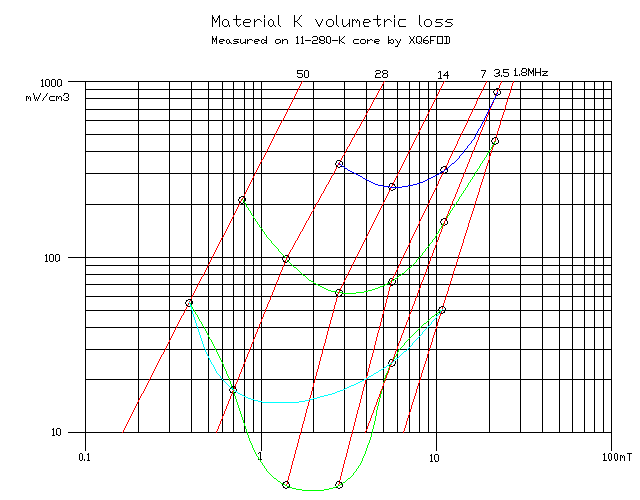Several years ago I received a gift from a friend and fellow ham, whose health was failing to such a degree that he knew he wouldn't ever again need the ferrite cores and other parts he had stocked. Nando, rest in peace. His gift included a nice assortment of magnetic cores, among them some "Ferronikits", which are kits of many cores of different sizes, of a specific material and type, made by the company Ferronics. Among these kits is one of material K binocular cores, and another of material K toroids. I used one of the largest toroids to measure its loss.

Material K is something special: A cobalt/nickel/zinc ferrite. It has the same initial permeability as Fair-Rite 61, but thanks to a small cobalt oxide addition and special processing it has exceedingly low loss. The shape of its basic loss curve is similar to that of material 61, having a pronounced loss dip, in this case more toward 14MHz than 7MHz. Unlike 61, material K has higher loss on 50MHz than on 28MHz - but still lower than 61! Ferronics K is the lowest loss material I tested.

The two lowest measurement points are so low that they are in a range where my test setup suffers from severe resolution problems: The temperature rise was just 0.2°C, and my thermometer has a resolution of 0.1°C, so the resolution-induced uncertainty is 50%! In the interest of scientific honesty, I drew those points anyway - but don't trust them. I drew the light blue loss curve that leaves out these two suspect points. But I think that the actual loss curve probably lies about halfway between the light blue and the lower green one.

On four frequencies I also had to make additional measurements at twice the normal "high" drive level I used, to be able to get data for the volumetric loss range that causes significant warming. That's the dark blue curve. No other material could be driven to such a high flux density on those frequencies without overheating in a matter of seconds.

If you can get Ferronics K cores, use them.

Note that the material K cores sold by Amidon are not this Ferronics K material. They are rated at an initial permeability of 290, and I understand that they are made by yet another company. I didn't test Amidon K material, because I don't have any of it. It would have been interesting to test it.


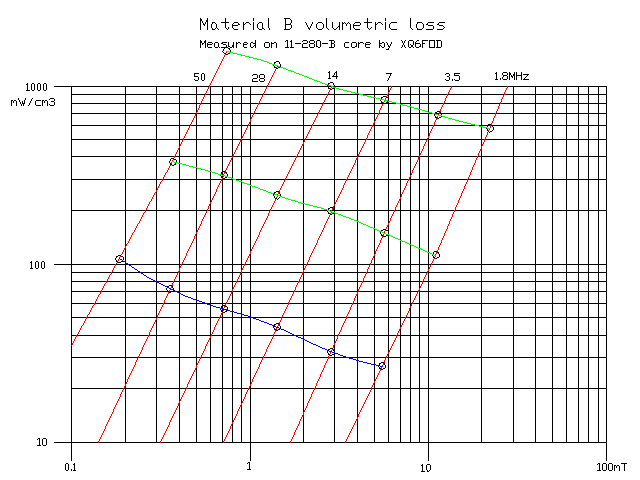Another of the Ferronikits I got from Nando contains toroids made from material B. That's not an RF material. It's a high permeability manganese/zinc ferrite, made for low frequency pulse transformers and the like. Just for comparison, I tested the largest toroid of this kit, which is the same size as the material K toroid I tested.

The characteristics turned out totally different from RF materials. While the loss on 1.8MHz was roughly in the same range as that of RF materials, the loss of material B  increases with frequency, at constant drive level, while in all RF materials it decreases. So the loss of material B in the high HF range and on 50MHz is very high.  So high in fact, that I had to run a third set of measurements, at an extra low drive level, to find out the loss in the range one could actually use this material. That's the dark blue curve.

At sufficiently low drive levels, even this material could be used at RF, but there is no reason to do so, since material 31 is available, provides a high permeability, and much lower loss.

Note that I always have 1.8-54MHz applications in mind. Instead if just one band, or a a few bands, need to be covered, matters can change a lot. In a pure 1.8MHz application, for example, material B would be a good choice.
 
Given that I was already measuring non-RF materials, I also started measuring the loss of a material 77 core. This is the main material sold by Fair-Rite and Amidon for power applications in the 20-100kHz range, typical for switchmode power supplies. It's a manganese/zinc ferrite having an initial permeability of 2000. Some hams do use this material in RF applications. But my measurements turned out so bad, that I decided to stop testing it, and I didn't draw a graph. Suffice it to say that material 77 has similar loss characteristics as material B, its loss rising with frequency at constant drive level, but also the loss is much higher than for B!

Also some hams use material 73 in RF transformers. Unfortunately the only cores I have in material 73 are small wire beads. They are too small to let me accurately measure their loss with my setup, and also the diameter ratio of those beads is too high to produce reliable results. Magnetic flux crowds in the small-radius areas of a core, and when the diameter ratio is too high, this effect seriously impacts the accuracy of material loss measurements.

   
Having run out of materials to test, I decided to test some cores made of the same materials but in other sizes.  The 61 and 43 cores I tested first were of the same size, but the 31 core was slightly larger. Since I have some material 61 cores of the same size as that 31 core,  I tested it.

The result was surprising: While the loss curves have the same shape than for the smaller 61 core, the volumetric loss is significantly higher! This much difference cannot come from my measurement setup. It's clear that ferrites are not always very consistent, in terms of loss.

Material 61, like several other low permeability materials, can be damaged by excessively intense magnetic fields. The manufacturers warn about this, but don't say how much is safe! Exceeding the limit will increase both the losses and the permeability, according to the lterature. Anyway this core was taken fresh from the package for testing, so I'm sure that I haven't applied a strong field to it. But could it be that it was exposed to such a field somewhere else, before I got it?


Digging deep in my treasure chest, I found three cores of identical size, Amidon FB-1020, in all three most-used Fair-Rite materials: 61, 43 and 31. So I measured their loss.

The FB-61-1020 bead has loss curves very close to those of the first, smallest bead I tested. This was reassuring, as it suggests that there are no hidden flaws in my measurement method, that would make it overly size-sensitive. And it also suggests that my FB-61-6873 core has abnormally high loss.


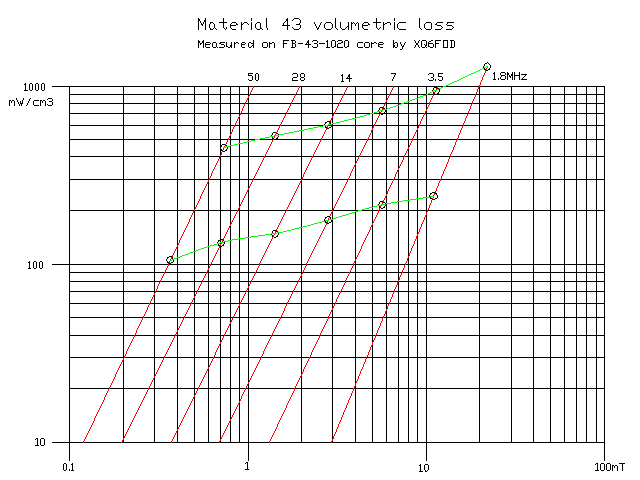The material 43 bead of the same size shows very much the same volumetric losses as the much smaller bead I tested first. This suggests that material 43 has better part-to-part consistency than material 61, but it's not a good idea to judge this from just two samples...


And the third in the group, the same-size material 31 bead, shows a loss close to that of its smaller brother, but not as close as the two 43 siblings. This suggests a theory: Within each family (manganese/zinc or nickel/zinc), the lower permeability members show wider dispersion of their loss characteristics. And this might be due by the low permeability being implemented by means of a distributed air gap (more filler, less ferrite in the final mix), just like in powdered metal cores, rather than by changing the basic formulation of the ferrites. But I'm purely guessing this.

The accuracy of these measurements

While I tried to work in a consistent, careful and repeatable manner while measuring the loss of these cores, the result shouldn't be considered to be highly accurate. There was always some heat loss through the test winding, some extra heat generated by copper loss in that wire, cotton isn't a perfect thermal insulator, the temperature sensor isn't perfectly coupled to the core and does conduct some heat away through its cable, and so on. In the very low loss range the resolution of my temperature measurements is poor, the oscilloscope used to measure the applied voltage only has a 100MHz  bandwidth, which means that at 50MHz it could already have a significant error, the scope probe is rated at 250MHz  but was used with its grounding clip pigtail, and so on. Despite all this, I think that my measurements are still usable, since the characteristics of ferrite materials themselves aren't highly precise. But one issue worries me more than the sum of all others: I didn't find really trustworthy, consistent information about the heat capacity of ferrite materials. I found values between 500 and 1250 J/kg/K published on the web, many of those sources don't say what type of ferrite is meant, nor even whether they mean ferrite as in magnetic cores, or bulk ferrites of various types. Narrowing it down from all I could find, I finally used a value of 750 J/kg/K for all my materials, but surely this is not precise. If the heat capacity of any of the materials is different from this value, the curves I drew for that material should be shifted according to that difference.  In case one material has a very different heat capacity than another, this might significantly affect the relative merits of them, making any hard conclusions drawn from my graphs a bit dangerous.

Ferrites vary their loss according to temperature. My tests were all done with the cores starting from my room temperature, about 21 to 22°C, and heating up at most to 45°C. So the loss measured is representative for normal "cool" operation, but not for cores that are running really hot.

Which material should you use?

Among the widely available materials (from Amidon and others), and considering only the ones tested here, 61 has the lowest loss, but also the lowest permeability. That's no news at all. What could be news to many people, is that the difference in practical use between materials is less than one would think! Although they can have a large difference in loss at a given flux density and frequency, the loss also varies at a very high rate with flux density. So, the flux density at which a core can operate, for a fixed amount of heating, varies only slightly between the various ferrite materials!

For example, let's assume that in a given application, with a given core size, a volumetric loss of 200mW/cm³ is acceptable. You now have to look at the graphs, and see at which frequency the ferrites have their highest loss, and see what flux density they can take at that frequency, for 200mW/cm³ loss. Let's do this now, for a 1.8-54MHz application. Here is the table I got:

Material K:  15mT at 1.8MHz.
Material 61:  12 to 14mT at 1.8MHz.
Material 43:  10 to 11mT at 1.8MHz.
Material 31:  9.2-11mT at 1.8MHz.
Material B:  0.23mT at 54MHz, thus roughly 7.3mT at 1.8MHz.

Let's forget about material B, which isn't an RF material. All the others fall in a range of 9.2 to 15mT, at 1.8MHz. That's an important difference, but not a really dramatic one! In a critical application you would want to get material K, if you can, and otherwise stick to 61, and see if you can get enough inductance from them at the low end of the spectrum. Instead in a less critical situation you would use any material you happen to have on hand, and just be sure to use enough turns per volt to keep the flux density low enough. Using more turns, or larger cores, reduces the upper frequency limit of a transformer, and that's why in a critical application you need to be careful with this. Equal-delay transmission line transformers (Guanella) give you a whole lot more headroom in this regard.

Note that the selection of material is dependent on the allowable volumetric loss. For example, let's assume that you will make a very small transformer. In these a much higher volumetric loss is acceptable, due to their higher surface-to-volume ratio, unless the insertion loss becomes a problem from the point of view of signal attenuation. Let's assume you can tolerate 1000mW/cm³. In this case, at 1.8MHz:

Material K:   28mT
Material 61:  20.5 to 23mT
Material 31:  20 to 22mT
Material 43:  20 to 21mT

Except for our clear winner, Ferronics K, the other three are pretty much in a tie!

And now let's go to the other extreme, when you have large cores, no forced-air cooling, and continuous operation, so that you can only accept 50mW/cm³ of core loss:

Material K: 10mT
Material 61: 8 to 9mT
Material 43:  5.7 to 6.2mT
Material 31:  4.8 to 6mT

Material K wins again, but 61 is close, the other two falling way behind. Note that both K and 61 are actually limited by the high frequency end, having slightly higher loss there than at the low end, at this low drive level.

And if you have an application that only needs a smaller frequency range, things change again.

This entire page is just about the loss of ferrite cores, but core loss is just one of several things you need to keep in mind when selecting the best material for a given application, and then select the shape and the size of the core. Here are some other considerations:

Initial permeability: The materials tested here vary between 125 and 5000. That's a huge range! Some people think "the bigger the better", other's think "enough is enough", but few realize all the implications of using materials of different permeabilities. Firstly, it's important to understand that the permeabilities really vary only in the lower frequency range. By 50MHz all of these ferrites have nearly the same permeability. Also at the low end of our frequency range the differences are already smaller than the very low frequency initial permeabilities suggest:

K: 125, highly inductive
61: 135, highly inductive
43: 550, with significant resistive component
31: 1100, with high resistive component

Note that these are the initial permeabilities, that is, the ones the materials show at extremely small flux density. At higher drive levels they change - specially the resistive component grows much larger. This effect is stronger in nickel/zinc ferrites than in manganese/zinc ones - you can see it by comparing the steepness of the 1.8MHz loss lines of 61 and 31 ferrites. That of 61 is much steeper.

A higher permeability can save the day, which is why many people love material 31. But it can also be a big liability. For example, when there is any net DC present in the windings. At a given core size, turns number and DC flowing in those turns, the DC flux density in the core is in direct proportion to its very-low-frequency permeability. Said clearly, a 31 core will have to carry 11 times as much DC flux as a 61 core! Very often this is critical in power amplifiers. While a 31 core would be totally saturated by a given DC, a 61 core might handle it comfortably.  With low permeability ferrite you can often just forget about imbalance currents in bifiliar chokes, for example - they typically won't cause trouble. Instead with high permeability ferrite even a small imbalance in a push pull amplifier might saturate the core!

Also an excessive permeability extends the response of a transformer down into an uneeded, and often unwanted, frequency range. It's typically an aid to amplifier stability to use transformers that have just enough bandwidth.

This extension of frequency response can be a real problem, when it extends so low that it reaches the higher components of the envelope signal, in transformers or chokes that carry a DC supply. In that case distortion can be caused, which manifests in splatter. So there are many good reasons to design transformers in such a way that they respond only over the desired frequency range, and not much beyond. And that often means that a specific permeability is optimal for a specific design. Not the highest one and not the lowest one, but the right one.

Another consideration is thermal effects. When a core runs warm, it changes its characteristics. In an extreme case, it can reach its Curie temperature, and at that point you no longer have a magnetic core, and the amplifier blows up! Material 43 has a Curie temperature of only 135°C.  Material 31 is even lower! Instead material 61 and material K both have it above 350°C, so it will never be reached in any normal circuit. Now you might say that 130°C also won't ever be reached. Be careful about that, though. Ferrites are poor thermal conductors, and the temperature inside the core can get much higher than that on its surface! The larger a core is, the more severe this effect becomes. So, in applications that might run very hot, you might be forced to use a material that has a high enough Curie point.

Cost and availability are powerful factors in deciding what core to use. Materials 61, 43, 31, and a few more, are widely available through Amidon and a few other distributors. Some amplifier builders have used Laird 28 material, which is available from some mainstream parts distributors. It has slightly lower permeability than 43 and might be a good choice, but specifications given by Laird are very thin. The complex permability graph they publish suggests that the material is less lossy than 43, but that's just the initial permeability, and it says little about the loss at higher flux densities. I don't have any Laird 28 cores here, so I can't test them. The same happens with cores from manufacturers like TDK, Ferroxcube, Kaschke, and several others. As long as I can't find a place where I can order them online, paying by Paypal or the like, and have them shipped to me by mail for a reasonable charge, I can't use those cores.

The cost of material 43 and 31 tends to be the same, while 61 is more expensive. That's a good reason to avoid 61when you can. On the other hand, in many circuits the cost of ferrite cores is small compared to that of other parts, such as high power transistors. This makes it reasonable to choose the best cores, regardless of a few dollars difference in their total cost.

Saturation flux density is of no importance in pure RF work, because a core will dramatically overheat at flux densities far lower than its saturation level. The exception could be pulsed operation at a very high peak-to-average ratio, but I'm writing this page mostly for homebrewing radio amateurs, and we hams don't use such narrow pulses.  But always keep in mind the problems associated with DC. If there is any chance that DC will flow in a winding, you need to evaluate what effects it will cause. A material combining high saturation flux density with low permeability tolerates the highest DC drive. But don't forget that materials like 61 and K are degraded by high flux density, and we are not told how much is safe!
  
A minor point to keep in mind is the electrical conductivity of the materials. Generally nickel/zinc ferrites are essentially good insulators, while manganese/zinc ferrites are bad conductors. If you use any manganese/zinc ferrite, including material 31, you need to keep in mind that it is slightly conductive, and insulate it where needed.
 
Life has never been easy. And ferrite cores are worse than life in that regard.

 
Back to homo ludens electronicus.